More on Representation
Timothy Boerst
My first exposure to the Rule of 3 was through reformed calculus materials as a student in a calculus courses at the University of Michigan. As I explain in more detail (see Representations in the Rule of 3), the Rule of 3 was a term coined to refer to three types of interrelated representations that could be quite useful when engaging in mathematical learning (exploration, communication, reasoning,…). Over the past few years I have used the Rule of 3 to formulate approaches to (and ways of thinking about) mathematics teaching. In a way representations used in my classroom support and are evidence of the interactions between students, mathematics content, and the teacher (see Representations in Teaching and Learning). This website is dedicated to supporting the understanding of the Rule of 3 and issues related to its role in teaching and learning fifth grade mathematics.
Representations in the Rule of 3
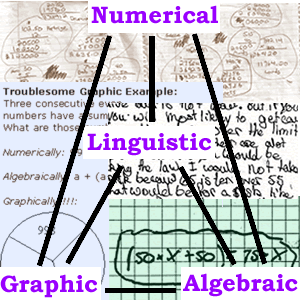
This collage shows the various sorts of Rule of 3 representations that were commonly used in my fifth grade classroom. The top is an example of extended student engagement with specific values in a numerical representation. The lower right hand corner shows an algebraic equation generated to encourage a more efficient search for a problem solution. The lower right hand corner portrays one of many spatial, pictorial, geometric, or other visual displays that fall within the graphic representation category. The centrality of linguistic work is highlighted in the diagram (written here, but oral also played a key role). Classroom sharing and understanding of the use of the numerical, algebraic, and graphic representations that comprise the Rule of 3 was substantially supported via linguistic means. More on defining the Rule of 3
Representation in Teaching and Learning
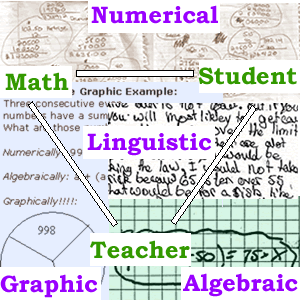
This collage also holds evidence of the ways in which Rule of 3 representations support interaction of teachers, students, and content that are at the crux of learning. The top of the graphic shows an example of the numerical representation that students routinely utilized when solving problems. While numerical representations did vary in appearance, students were quite comfortable engaging in mathematics with specific values in mind. The algebraic equation in the graphic was one that a student and I generated to test a series of values that may or may not have been solutions. Written algebraic expressions were usually formulated in joint efforts such as this. Thinking about fifth grade math through Rule of 3 representations sometimes pushed my own mathematical thinking as illustrated in my experimentation to find graphic representations that would be of use in solving particular problems or communicating ideas about solutions. Strategically located at the center is an example of linguistic work, the kind that often allowed me a window on the status of the interaction between students and the mathematics they were attempting to learn. In this way linguist representations often provided a means of bringing together all three elements of the triangle.